Mastering Quadratic Factorization: Common Challenges and Effective Strategies
Published October 19, 2023
Learning to factorize quadratics is a crucial skill in algebra, but it's not always a straightforward process. Students often encounter various challenges that can hinder their progress. In this blog post, we'll explore some of the common hurdles students face when tackling quadratic factorization and discuss effective strategies to overcome them.
- Recognizing Patterns: The Initial Hurdle
One of the primary challenges in factorizing quadratics is recognizing the patterns within the expressions. This is especially true when coefficients differ from the standard quadratic format ax^2+bx+c. Encouraging students to develop an intuitive sense for identifying these patterns is crucial.
Strategy: Visual Aids and Practice. Using visual aids like diagrams and graphs can help students grasp the relationships between different terms in a quadratic expression. Additionally, consistent practice with a variety of quadratic equations can help reinforce pattern recognition skills.
- Understanding the Factorization Process
Understanding the step-by-step process of factorizing a quadratic equation can be complex, especially for beginners. It involves finding two numbers that multiply to give a constant term and add up to give the coefficient of the linear term.
Strategy: Break It Down. Break down the factorization process into smaller, manageable steps. Emphasize the importance of finding pairs of numbers that satisfy the conditions and guide students through this problem-solving process.
Play 'Wrecks Factor' on Mangahigh

- Handling Negative Numbers
Negative numbers can add an extra layer of complexity to factorization, causing confusion for some students. Ensuring a solid grasp of basic arithmetic operations with negatives is essential.
Strategy: Conceptual Understanding. Encourage students to view negative numbers as mathematical tools rather than obstacles. Provide real-world examples that demonstrate the application of negative numbers in factorization.
Play 'Wrecks Factor' on Mangahigh
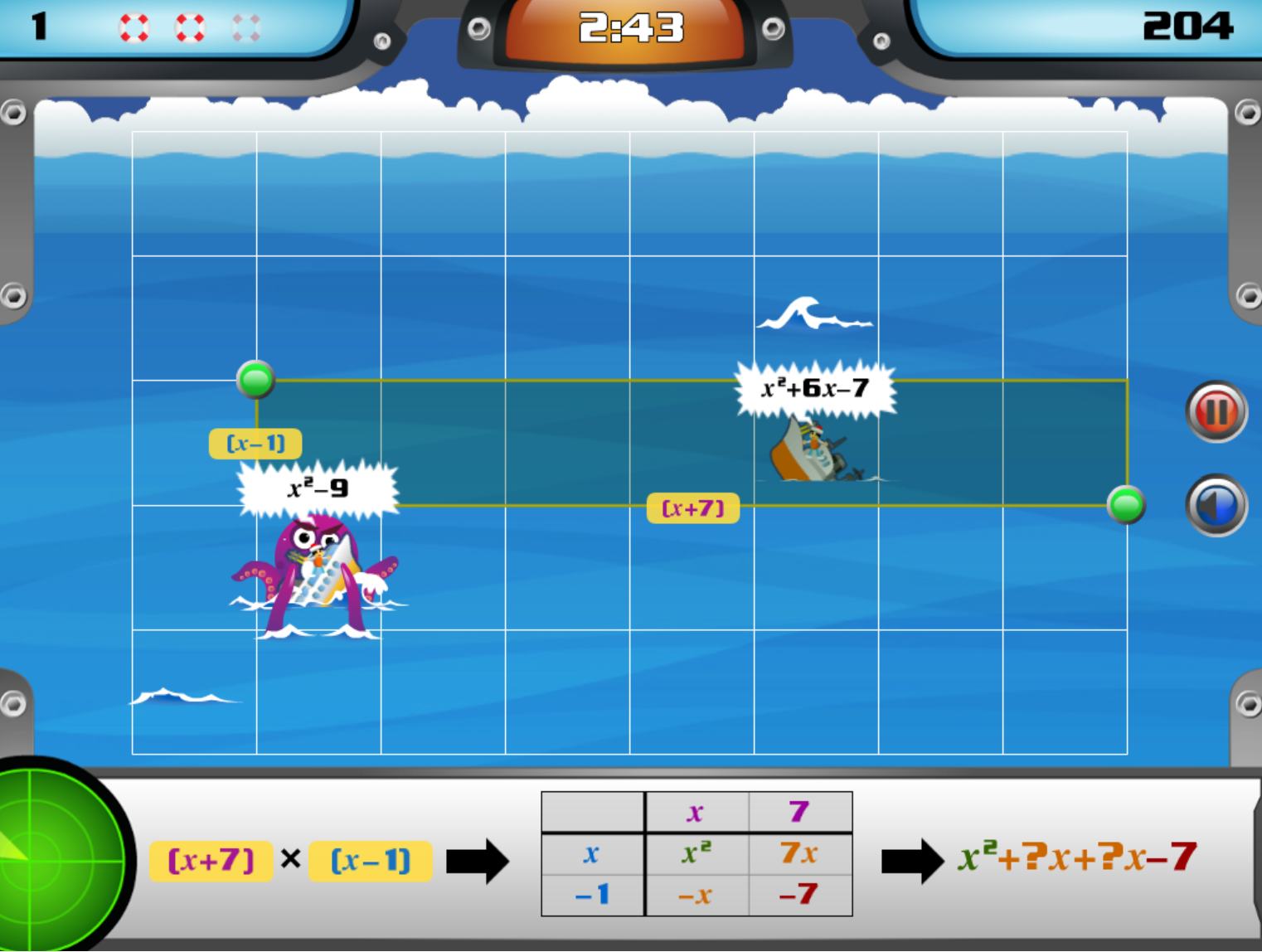
- Factoring with Coefficients other than 1
Dealing with quadratics where the coefficient of x^2 is not 1 requires additional steps and can be more challenging.
Strategy: Practice with Variations. Introduce quadratic equations with varying coefficients to gradually build confidence in factorizing non-standard forms. Emphasize the importance of adjusting the factorization process accordingly.
- Practice, Practice, Practice
Trial and error is inherent in the factorization process, which can be frustrating for students lacking confidence in their mathematical intuition.
Strategy: Encourage Persistence. Reinforce the idea that making mistakes is a natural part of the learning process. Provide ample practice opportunities and offer constructive feedback to help students build confidence and improve their factorization skills.
- Application in Real-World Problems
Translating word problems into quadratic equations for factorization can be a stumbling block for some students.
Strategy: Contextualize Learning. Present real-world scenarios that require quadratic factorization and guide students in formulating the corresponding equations. This approach helps bridge the gap between abstract concepts and practical applications.
Mastering quadratic factorization is a valuable skill that lays the foundation for more advanced algebraic concepts. By acknowledging and addressing the common challenges students face, educators can create a supportive learning environment. Through a combination of clear explanations, ample practice, and real-world applications, students can confidently navigate the intricacies of quadratic factorization. Remember, persistence and a growth mindset are key to overcoming these hurdles and achieving success in algebra.
